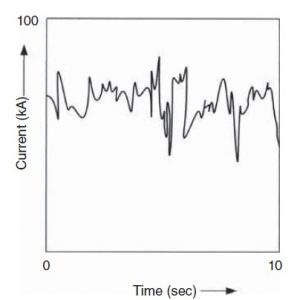
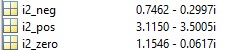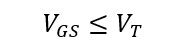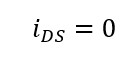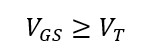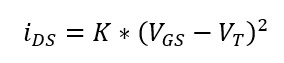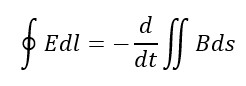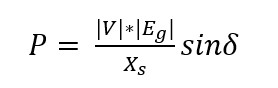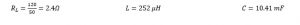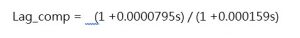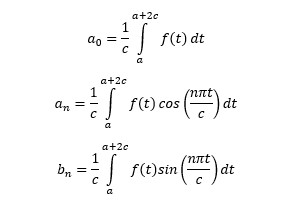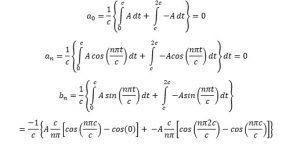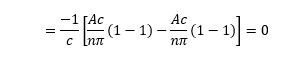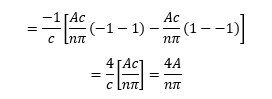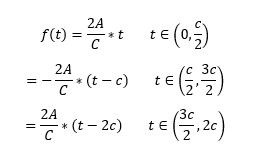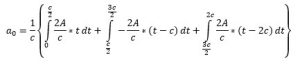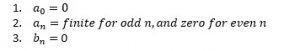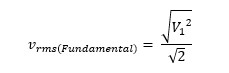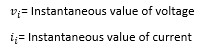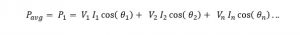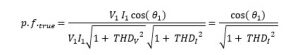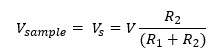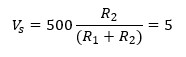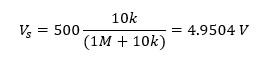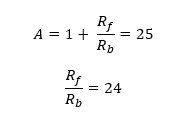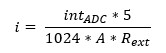In electrical engineering, we almost always overthink “Blackouts”!!!
Bear with us for the little Background story.
In the summers of 2019, CEV Aantarak began studying Blackouts, namely the Indian Blackout of 2012 and the Ukrainian Blackout of 2015. That time we didn’t get too much of technical details, rather just getting the things on periphery of the event, by studying the reports of CEA, POSOCO and other concerned authority, until one of team member Anshuman Singh secured a research intern at IITKGP to study the exact phenomenon which triggered that largest blackout of the history, the 2012’s. Sir carried out his preliminary works and finally got to put up his great work in the blog Fault Analysis in Power Systems. The approach which he and his colleagues used to solve the problem was indeed a decent one, however, due to the inherent glitches in that particular protection philosophy itself, it didn’t fix the problem completely. And finally, now in 2021, we moved one more step ahead by studying the technology which addresses that old doomed problem, “zone 3 maloperation of distance relay due to load encroachment”, and more importantly “the drawbacks of conventional SCADA system”.
Recap: What was Zone 3 Maloperation of Distance Relay?
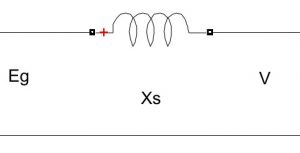
When any kind of fault occurs in any component of power system, what basically happens is that a high potential point gets connected to a low potential point (typically ground) via a very small resistance path, leading to flow of dangerously high current by virtue of Ohm’s Law, and thereby dissipating great thermal energy as indicated by joules law, or i^2r.
All components, especially high voltage systems must be protected against this possibility. Transmission lines obviously subjected to the external environment are most prone to faults.
Relaying is what technically called arrangement to protect against the destructive effects of faults. Based on economy and other factors like accuracy and fastness various types of relaying schemes are employed.
Recall the consequences of fault:
- Large current
- Small impedance (resistance)
Based on these two criteria we have an overcurrent relaying scheme and distance relay scheme respectively. So, when the current goes beyond a certain threshold or when the impedance goes below a certain threshold, the scheme correspondingly generates (or issues) a trip command to Circuit breakers to open up and isolate the faulted point from the healthy system.
For strategically significant lines distance relay is technically superior to overcurrent relay.
A distance relays works by categorizing its area of operation into three zones. This is done in order to provide backup protection by introducing increasing time delays for successive zones.
However, distance relay also has its own limitation.
The most prominent of them is its maloperation under heavy load conditions.
The relay misidentifies the fault when the line is heavily loaded and as Anshuman explained losing a line when it is heavily loaded is seriously fatal. (Hint: leads to cascaded tripping). In simple language distance relay works on the principle of sensing the impedance and operating when impedance falls below a threshold. Increasing loading is also manifested as decreasing impedance of system (Analogy: smaller is the value of resistance more is power dissipation for a given voltage level), thus causing the relay to trip the CBs.
This is a zone 3 maloperation of distance relay due to load encroachment.
Anshuman’s Solution in Short and problem with solution
The distance relay, unfortunately, is not blessed by his masters, i.e., the EEs, with the intelligence to distinguish the fall of impedance due to increased loading or due to a genuine fault. The relay is more like a Pharmacist who gives paracetamol to anyone having a fever.
Anshuman and his team demonstrated a procedure, which though is certainly a viable economical method to avert an impending blackout however is not so all-in-one fix and consumer-friendly.
Fault Analysis and related Technical problems in Power System
It is basically directly addressing the cause which is causing a drop in impedance, i.e., the increasing active power consumption. So, the idea is to drop some quantum of the load off the grid to stop the impedance from further dropping.
This leads to an implementation question.
There are hundreds if not thousands of buses connected to a transmission line end. So, the load shedding at which bus shall be performed, in order to achieve a certain increment in impedance for a minimum amount of load shedding and also the considering the fact that we don’t push the buses into voltage instability.
However, the issue that remains unresolved by this approach is quite obvious.
The mathematical answers that we get from the algorithms may not be practically feasible. That is this approach does offer the method to distinguish between the VQ sensitivity of buses but doesn’t take into account the criticality of buses i.e., a hospital is connected or a night irrigation facility.
Apart from this Zone 3 Maloperation problem, we have another setback that significantly threatens the security of the power system in general, called the conventional SCADA (Supervisory Control and Data Acquisition Systems), which quite contritely is deployed to provide control over the large grid operations.
The Inherent Problems of conventional SCADA systems
- No measurement of voltages and current phase angles: This problem can be understood better in terms of another question.
What is the phase of this signal?
A trash question, phase is a relative quantity and thus, we need to define a reference first.
Undoubtedly the measurement of angles of voltage and current phasor in power system which rotates at a rate close to 50 Hz or 314.6 rad/sec, requires a reference. Considering, the vastness of the landscape over which PS is spread it becomes a technically challenging task to provide the same reference to all the locations. This makes the unavailability of the angular separation between bus voltages and limits the ability of operator to get the true nerves of the system (i.e., the transient stability).
- Time skew between measurements: RMS Voltage measurements made using SCADA even have no common time reference, hence one has no means to differentiate whether data coming are made at same instant or not.
- Low update time i.e., large scan cycle time: with the methodology it takes around few seconds to few minutes to get new values of variables, so the operator lags the systems by about a few seconds or few minutes, hence no real-time system awareness. It is exactly like a MARS mission, where you get to know about the touch-down 12 minutes later, as light travel at finite speed and delay generated by the communication equipment, only difference is power system engineers have much more wide options to trigger some preventive measures to avert a catastrophe if they get system parameters on time.
- Stringent requirement on Control Center computational capabilities: since the data streaming has so many uncertainties, to extract the useful data and figure out the true condition of the power system puts a challenging task to computers. All these problems are more severe and serious than they sound. The North American blackout of 2001 and the European blackout of 2003 were results of the foggy image that the SCADA presented to the control center. The investigative task force committees independently recommended the use of Synchrophasor technology in real-time monitoring of the system, which back then was only used in small numbers to store data and conduct post-event analysis.
The Synchrophasor Technology
The inability to do phase angle measurement as well as time skew and slower update rate of Voltage measurements were prime setbacks of the SCADA system.
Synchrophasor technology comes to address those problems. This method of measurement is significantly advance than the conventional SCADA system. The Synchrophasor measurements provide following services:
- Measurement of RMS bus voltages and current along with phase angle wrt to a common reference signal shared by the whole power system.
- No time skew: all measurements voltage magnitude, phase angles, frequency are also time-synchronized and are even time stamped
- High-speed update rate: from 25 samples to 50 samples per second depending on PMU devices: all these lead to give operators the wide-area situational awareness in real-time, and enables them to take much better decision to shred load or generation, trip a CB, direct the line flow, add C-banks, etc.
- Accurate measurements thus significantly lower computational requirements for state estimators.
The Idea of Phase Angle Measurement: Using the GPS signals
We saw the need for a common reference signal as inevitable for phase angle measurement.
This system however depends quite heavily on two things:
- Accuracy of common reference i.e., the GPS clock
- Communication systems reliability
The GPS provides one pulse per second at all the locations spread over the entire peninsula. The pulse received simultaneously by all the measurement units triggers them to begin their measurement, wrt to an imaginary zero phase sine wave reference.
So, a GPS receiver is required.
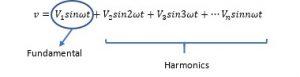
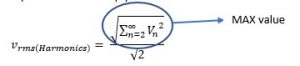
These measurements to be made successfully require stringent requirements on the waveform to be measured itself. A waveform having harmonics will lead to significant errors.
So filtering is required.
Also, the kind of mathematical operations required to be made on signal requires it to be in represented in digital equivalent.
So, analog to digital converter is required.
Fourier transform can now be carried out on digital samples, using a commonly available economical microprocessor, to yield the magnitude and what we can say absolute phase angle.
So, a microprocessor is required.
The data contained in a GPS has incredible amount of other useful data, including the time and date, location coordinates, etc., which can now also be stamped with the power systems measurement to be sent to the control center.
So, a secure, reliable, and fast communication terminal is required.
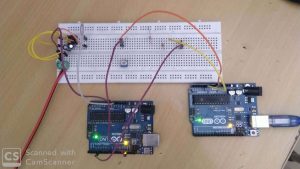
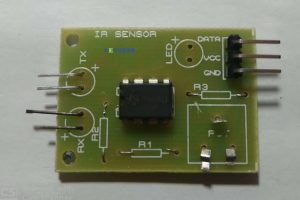
The PMUs: Device that executes the idea of phasor measurements
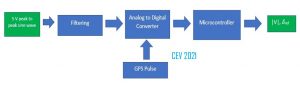
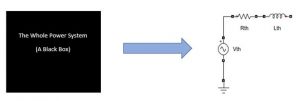
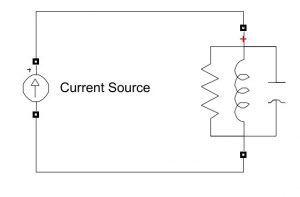
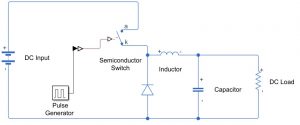
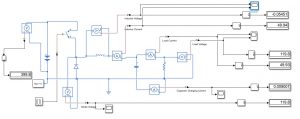
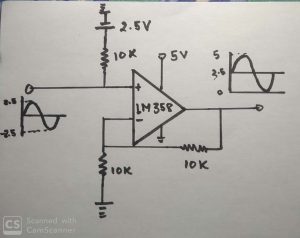
The Basic Schematics:
Components blocks:
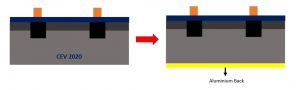
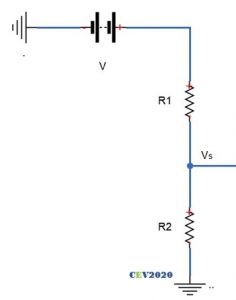
We have extensively described and executed 1st stage conversion on how to obtain 5 V peak sine wave from 230 V mains supply in many previous accounts.
In power systems where voltage levels are of the order of hundreds of kV and current in kA, the potential and current transformers are used to step down these values.
- Filtering:
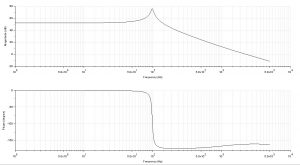
It is said that in analog engineering 90% of the stuff is just filtering, 9% amplification and the rest 1% is other nuts and bolts. That gives quite a clear-cut indication of fact that how much essential filtering is. Filtering is our first defense against errors.
Errors in various kinds of signal are generally indented by their characteristic frequency finger-prints. For general power systems the signals of interest i.e., the voltage and the current signal meander in a narrow range band of 49.5 to 50 Hz. So, a low pass filter is appropriate to stop most of the measurement noise, in the signal. Practically a filter is implemented by active and passive components.
- A/D Converter and the GPS:
Here at ADC the core PMU function is operated. The key difference between the SCADA and the PMU system is the availability of common time reference at all the terminals. So, all the ADC, every time, begin their measurement at the same instant and thus the information required to find the relative phase displacement between that signal is captured.
- DFT:
Once we have got a faithful digitized replica signal of analog version of voltages and currents, we enter a comfort zone, by the virtue of powers offered by a modern computing platform like a microcontroller. Instead of building physical circuits using passive components, we simply write down our mathematical tricks in a precise language (Programming Language as they call it) and print it on uC and we are done.
Here in MATLAB, we are rescued by already available setups to perform DFT. We have both Simulink block as well as in-built function.
FFT function description:
The “fft” MATLAB’s inbuilt function that generates an output vector [1*n] consisting of complex data points for an input of discrete time-domain signal having n samples.
Equivalently it generates n number of what is defined as bins, each having a corresponding magnitude and the phase angle value. This is essentially frequency domain representation of the input signal, as each bin corresponds to some corresponding frequency, depending on sample frequency and length of signal.
Now the magnitude and phase angle of a particular bin related to actual magnitude and phase angle of corresponding frequency in a defined way as follows:
 where x[n] is magnitude of bin number n.
where x[n] is magnitude of bin number n. The point to be noted here is we were working in Simulink till now but to apply FFT we typically wrote a script in m-file. This one of the greatest advantages of the MATLAB platform. All of the stuff we do in the simulation is to be implemented practically, so each component has a corresponding hardware counterpart. For CT, PT electrical systems made of copper and iron (loosely speaking), ADC, and GPS receiver are implemented by dedicated integrated circuits, and for signal processing and data visualization, we use microcontrollers, which are operated by the brunt codes. The former is executed in Simulink and later is exactly mimicked by m-file very conveniently.
The point to be noted here is we were working in Simulink till now but to apply FFT we typically wrote a script in m-file. This one of the greatest advantages of the MATLAB platform. All of the stuff we do in the simulation is to be implemented practically, so each component has a corresponding hardware counterpart. For CT, PT electrical systems made of copper and iron (loosely speaking), ADC, and GPS receiver are implemented by dedicated integrated circuits, and for signal processing and data visualization, we use microcontrollers, which are operated by the brunt codes. The former is executed in Simulink and later is exactly mimicked by m-file very conveniently.
The block used to import data is “To workspace”.
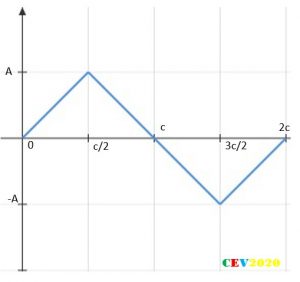
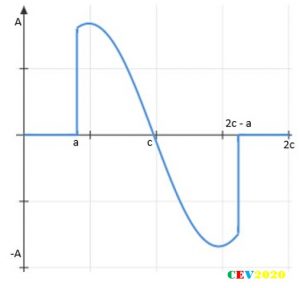
Windowing and Zero padding: When we take samples of voltage and current waveforms using ADC and if the no of waves captured is not integral in number, then we get what is called as spread of frequency spectrum, which can be seen in terms of side lobes around the central lobe. This leads to the decreased measured magnitude of the fundamental, i.e., error in measurement. We can manage to get integral no. of cycles of measurement by essentially fixing the time for which ADC collects sample of a 50 Hz sinusoidal waveform, however, in the practical world, the frequency never settles at 50 Hz and tend to meander around 50 Hz (in range of 49.5- 50.5 Hz) as a result of disbalance in instantaneous real power generation and consumption. This in turn causes a non-integral no of waves to be captured. To deal with this, a typical hannowing window is applied to the ADC digital output signal to compensate for the trailing edges of the signal.
%% PERFORMING FFT on Bus 1 % Sample frequency fs= 20000; %Storing phase V of bus 1 b1vR= out.b1vR; % Pre-signal Conditioning %to improve the fft accuracy for non-integral waves v1r= b1vR'; v1r = v1r.*hanning(length(v1r))'; V1R = [v1r zeros (1, 10000)]; % Performing FFT V1R = fft(V1R); % Obtaining the magnitude and phase values for Bus 1 V1R_mag = abs(V1R); V1R_phase = angle(V1R);
Notice the absence of any side lobes in the pre-conditioned signal.
- Sequence Analyzer:
What we have after FFT is magnitude and phase information of each phase at each bus. When unsymmetrical faults occur in system, we get unsymmetrical phase voltage magnitude readings as unsymmetrical faults lead to unsymmetrical currents and hence unsymmetrical voltage drops in generator and transformer windings and thus unbalanced voltage at the buses. This data cannot be used to effectively to interpret the system, leave aside the detection of fault and tripping correct circuit breakers. CL Fortescue is his ground-breaking mathematical work showed us an effective way to deal with unsymmetrical systems. The unsymmetrical components can be resolved into three sets of balanced components. Based on those components the faults can be identified by their characteristic resolutions.
%% Sequence Analyzer for BUS 1 %Define alpha and alpha squared a = -0.5 + 0.866*i; b= a*a; %Define sample frequency and max bin number N = length(V1R); fs = 100000; z= 2/N; bin_max = 10; %Phase Voltage vector representation vrb_1 = 0.37792*z*V1R_mag(bin_max) *(cos (V1R_phase(bin_max)) + i*sin (V1R_phase(bin_max))); vyb_1 = 0.37792*z*V1R_mag(bin_max) *(cos (V1Y_phase(bin_max)) + i*sin (V1Y_phase(bin_max))); vbb_1 = 0.37792*z*V1R_mag(bin_max) *(cos (V1B_phase(bin_max)) + i*sin (V1B_phase(bin_max))); %Sequence analyzer v1_pos = 0.3333*(vrb_1 + b*vyb_1 + a*vbb_1); v1_neg = 0.3333*(vrb_1 + a*vyb_1 + b*vbb_1); v1_zero = 0.3333*(vrb_1 + vyb_1 + vbb_1);
- Data visualization:
%Bus 1 Voltage plotting
bin_vals = [0: N-1];
fax_Hz = bin_vals*fs/N;
N_2 = ceil(N/100);
subplot (4, 2, 1)
A = 0.37792*z*V1R_mag;
plot (fax_Hz (1: N_2), A (1: N_2))
xlabel ('Frequency (Hz)')
ylabel ('RMS in kV');
title ('Bus 1 Phase Voltage - R Phase');
Apart from visualization of waveforms in time and frequency domain, we built a GUI to help see and comprehend the RMS magnitude, phase angle information, frequency and Circuit breakers status is more easy and convenient way. The app designer application of MatLab is used to build the GUI in graphical mode and then automatically generate its m-file to be embedded within the main code.
How it solves Zone 3 Maloperation?
Distance Relay works on the principle of impedance measurement. For a measured value of impedance less than the set value the relay issues a trip command. For zone 3 the relay maloperate as the measured impedance reduces below a threshold value either due to fault or even in cases for overloading (fanatically called load encroachment). Ideally, the distance relay shall operate for the first case but not for the second case. However, there is no true way to differentiate between the two, unfortunately, we had to go for load shedding, which just tends to avoid the locus of the impedance seen by relay to entering from zone 3.
Notice that the zone 3 protection is backup protection, thus operates with a time delay of 1 second. Now, this backup protection responsibility can be given to PMUs. Since there will always be a communication delay which is of the order of few milliseconds, so it cannot replace the instantaneous primary protection provided by distance relay. However, by measurement of voltage and phase angle, we can very well distinguish between the fault and overloading, this distinction is strictly not required for primary protection.
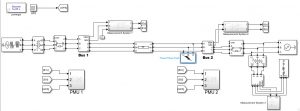
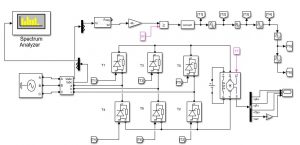
The Backup Protection by PMU: A two bus testbed
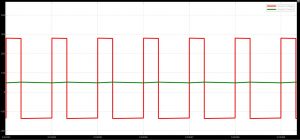
A simulation of three-phase bolted faulted at the bus 2.
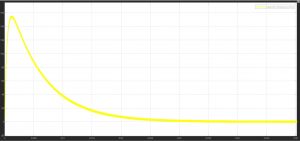
Unbalanced current depending on the instant of fault a particular follows the highest peak. As expected, an increase in the current due to the fault, since the fault is symmetric hence the fault current settles to a balanced set steady state.
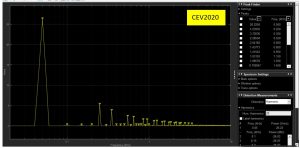
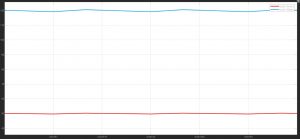
The frequency-domain information of current and voltages by PMU shows the presence of frequency other than fundamental, 50Hz. Time-domain representation accurately captures the R-phase fault current and voltage.
Also notice the presence of significant magnitude of negative and zero sequence voltages and currents, giving a reliable indication of the faulted state of the system.
GUI Output:
Conclusion
This blog ventured to prove that the PMU data can be very effectively used to differentiate the faulted condition from a healthy or heavily loaded system. Unlike distance relay protection it provides reliable backup protection which is resilient towards load encroachment. And since PMU data takes few milliseconds due to communication delay it thus cannot be utilized for the primary protection.
Quite evidently all the usual SCADA problems are effectively handled by PMUs. Based on the availability of phase angle data, the angular separation data between the voltages of different buses gives much better visibility of the true state of system.
The applications of synchronized measurements are numerous in number and tremendous in their scope. The conventional ways of doing things like fault analysis, tripping events analysis, state estimation, grid monitoring, black start, etc. which were barely and insufficiently carried out by the SCADA system can be now done easily and accurately using synchronous measurement data. It has to be further noted that, after 30 years since inception, now being in the advanced stage of development PMUs are now being deployed for modern applications like renewable integration, voltage instability problems, highly complex grid monitoring and control.
References
- Fault Analysis and related Technical Problems in Power Systems: Anshuman Singh Jhala
- Power System Backup Protection in Smart Grid: Ms. SU Karpe and Prof. MN Kalgunde
- Synchronized Phasor Measurement and their Application, AG Phadke and JS Thorpe.
- Synchrophasor Initiative in India, June 2012, POSOCO-India
- Novel Usage of Synchrophasor for system improvement: POSOCO, New Delhi, India
Thank you!
Keep reading, keep learning.
TEAM CEV.